The Dredge Tank. For posting all the low tier reactionary bullshit that you can't post anywhere else. Got some bullshit from Reddit with 2 upvotes and want to share, post it here.
This community was created with the purpose that Rule 8 fans will just block it.
The rules are literally The Dunk Tank's rules, just without rule 8.
Rule 1: All posts must include links to the subject matter, and no identifying information should be redacted.
Rule 2: If your source is a reactionary website, please use archive.is instead of linking directly.
Rule 3: No sectarianism.
Rule 4: TERF/SWERFs Not Welcome
Rule 5: No ableism of any kind (that includes stuff like libt*rd)
Rule 6: Do not post fellow hexbears.
Rule 7: Do not individually target other instances' admins or moderators.
lesser evil calculus where it's literally just n compared to n+1 is so unhinged like, this is not a meaningful measurement. if the harris admin promised to kill 200000 and maim one where the trump admin promised to kill 200001 are we making a difference here? kill 200000 and leave one on life support? what are we doing here. it's so bleak 
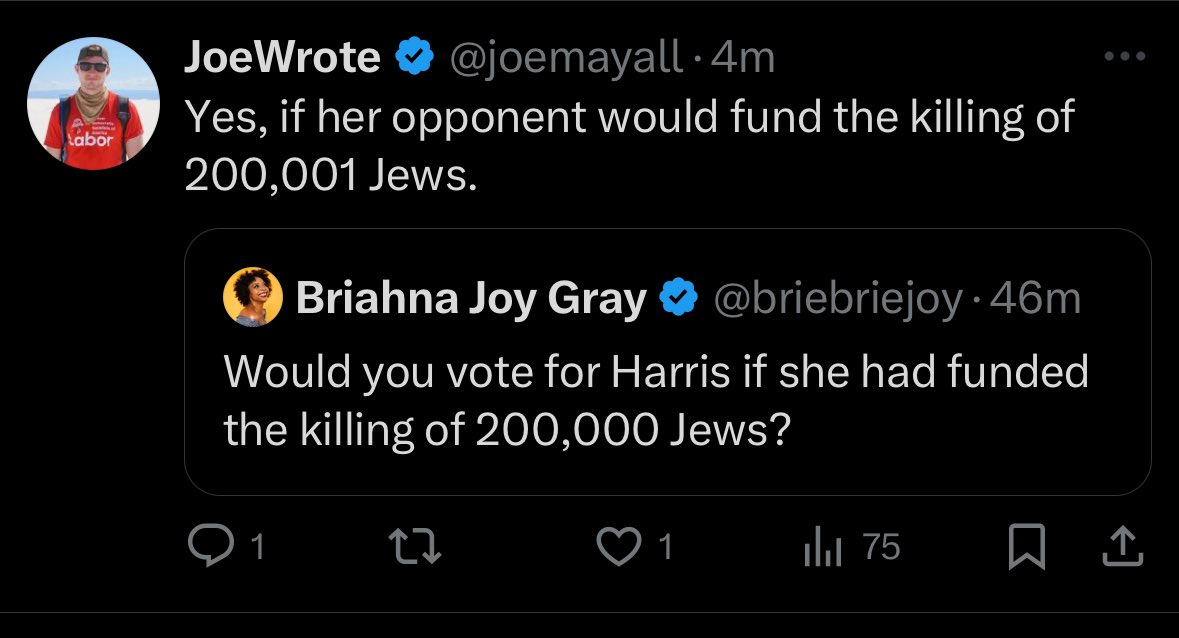
Theorem: If the difference between Harris and Hitler is less than or equal to 1/n for all positive integers n, then Harris is equal to Hitler.
Proof by contradiction: Suppose Hitler is strictly worse than Harris, then Harris < Hitler on the evil axis.
Then we have: 0 < Hitler - Harris < 1/n for all positive integers n.
Multiplying all sides by n: 0 < n(Hitler - Harris) < 1
Dividing all sides by the difference between Hitler and Harris: 0 < n < 1/(Hitler - Harris)
But that implies the set of positive integers are bounded above by 1/(Hitler - Harris). The set of integers are an inductive set, which is any set that contains the number 1 and contains x+1 for all elements x in the set. If the set of positive integers is bounded above by 1/(Hitler - Harris), then it must have a least upperbound b such that b <= 1/(Hitler - Harris). That means the set must contain some number k such that k > b-1 otherwise b-1 would be the least upperbound. But since this is an inductive set, it must contain k+1 if it contains k, and k+1 > b, proving the set of positive integers is actually unbounded and therefore Hitler = Harris.